The Englishman HARDY and the German WEINBERG could show that the frequency of homozygotes and heterozygotes in a population stays constant for generations if certain conditions are fulfilled. The HARDY-WEINBERG law permits the theoretical calculation of the frequency a certain genotype has in a given population independent of the number of existing alleles.
The Mendelian laws start out from two individuals (parents) and
their offspring. Hereditary traits as they have been described till
now can only be understood under controlled conditions. Ratios like
3:1 will hardly be discovered in nature since every species has to be
regarded as a group of populations in which certain genotypes occur
in certain amounts hard to capture. The frequency of an allele can be
very low and genetic combinations where it has part in will
inevitably be very rare.
The Englishman G. H. HARDY and the German W. WEINBERG showed independent of each other in 1908 and 1909 that the frequency of homozygotes and heterozygotes stays constant for generations, if
|
|
the population is very large, |
|
|
the individuals can pair without limitations (if they belong to different sexes and live at the same place and at the same time, of course), |
|
|
there is no selection of certain alleles, |
|
|
no gene migration occurs and |
|
|
no mutations take place. |
Their mathematical model went down in the literature as the
Hardy-Weinberg equilibrium.
given: two pairs of alleles, A and aassumed: the frequency of A shall be p = 0.9 (= 90%) that of a shall be q = 0.1 (= 10%)
from that follows: p + q = 1
In the population, the genotypes AA, Aa and aa will thus be found.
The produced germ cells would either contain A or a. If they cross
according to chance, it has to be taken into account that germ cells
containing A have the frequency p and germ cells equipped with a the
frequency q. These genotypes will accordingly occur in the following
generation with the following frequencies:
AA = 0.9 x 0.9 = 0.81Aa = 0.9 x 0.1 = 0.09
aA = 0.1 x 0.9 = 0.09
aa = 0.1 x 0.1 = 0.01
or, expressed mathematically: AA = p2 ; Aa + aA = 2pq ; aa = q2
or: p2 + 2pq + q2 = (p + q)2 = constant
Or, expressed in words: under the conditions mentioned above, the
original ratio of the alleles A and a will be retained from
generation to generation. There can be any number of alleles per gene
in a population. The genome of an individual is therefore just a
chance selection of the whole gene pool.
The Hardy-Weinberg law allows the calculation of the heterozygous individuals' frequency. When two alleles exist, it can never be larger than 0.5. The following picture shows the quantitative relation between the frequencies of the alleles and those of the respective genotypes (according to D. S. FALCOMER, 1960).
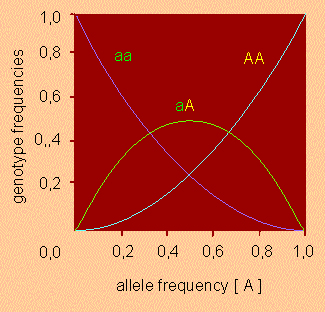
If an allele has a high frequency, the relation of the genotypes
will shift strongly in favour of the respective homozygous genotype.
But since the preconditions for Hardy-Weinberg are usually not given,
plant populations being often very small and self-pollination being
no exception, the law cannot be applied here. MENDEL himself tackled
this problem in his classic study in 1866 and asked how the splitting
ratios of subsequent generations would look, if the offspring of
every new generation would always be crossed with each other. He made
the following extrapolation:
|
|
|
|
|
relative values |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|||
|
" In the 10th generation, 2n-1 = 1023. There are accordingly 2048 plants that emerge from this generation, 1023 with the dominant and 1023 with the recessive trait but only two hybrids." |
|
|