1. 
2. Help
3. Log In

LESSONS FROM CLASSICAL THERMODYNAMICS

1) Define System as separate from Surroundings.

2) Identify Change in State that system undergoes (Initial and Final states).

3) Change in state occurs through a process defined by a pathway. Work (w) and heat (q) exchanged with the surroundings are dependent on the pathway. However, we can in principle find a pathway for which the change in state occurs (through appropriate coupling to the surroundings), in which the maximal amount of work (w_max) is done. Such a pathway defines a reversible process, and the heat exchanged is called the reversible heat (q_rev). A reversible process does not occur at a finite rate.

FOR A REVERSIBLE PROCESS W_max AND Q_rev HAVE FIXED VALUES.

Since we can in principle find a reversible process for any change in state, we can also define the fixed values for maximal work and reversible heat for any change in state.

The parameters, w_max and q_rev, which define the reversible process, are of special importance in thermodynamics, because, unlike the work and heat exchanged in an irreversible (or "spontaneous") process, they have unique values for a defined change in state; they are variables of state. Further analysis of the heat exchange led to the concept of entropy.

At constant T,

dE = w_max + q_rev (for a "reversible" process)

dE = dA + TdS (dS = q_rev/T , Entropy change; TdS is the reversible heat)

dA = dE - TdS (Helmholtz free energy change, or maximal work)

dG = dE - (-PdV) - TdS (Gibbs free energy change, or useful work)

dG = dH - TdS (dH = dE - (-PdV) , Enthalpy change)

dG = dA - (-PdV) (Useful work is maximal work minus the work done against the atmosphere)

The Gibbs free energy differs from the Helmholtz free energy by the work (-PdV) performed against the atmosphere, which is not regarded as "useful". The Gibbs free energy is sometimes called the "useful" work. For most biochemical processes, no significant change in volume occurs, and dA and dG have the same value.

5) Spontaneous process

During any process which occurs at a finite rate (a "spontaneous" process), the system loses some capacity for performing work. For all spontaneous processes, the change in state of the system must have a negative value for dG. The process must (in principle) be able to perform useful work on the surroundings, if a suitable coupling process is available.

6) Change in state by a "spontaneous" process does not occur by a reversible pathway. Work obtained is less than w_max and the difference is passed to the surroundings as heat (entropy increase of surroundings).

7) Conditions for spontaneous process

For System, change in state must have -dG

For System + Surroundings, entropy must increase (the entropy of the universe is increasing to a maximum)

8) Because dE, dH, dG, dA, dS are variables of state, they have fixed values for any defined change in state. From this it is obvious that if a process occurs in which the system is returned to its initial state, the values for changes in the variables of state are zero (the cyclic integral for dG, dA, dH, etc. is 0).

9) Because dE, dH, dG, dA, dS, etc. are variables of state, any defined change in state can be achieved by a series of changes in state. The values for changes of each variable of state can be summed along this series to give the overall value for the change.

State 1 ---------------------------------------------------------------> State 2

State 1----> State a ----> State b ----> State c ----> State d ----> State 2

dG_(2-1) = dG_(a-1) + dG_(b-a) + dG_(c-b) + dG_(d-e) + dG_(2-d)

10) A change in state of the system can occur which involves many different sorts of work (mechanical, pressure-volume, chemical, electrical, electrochemical, etc.). In principle, we can imagine a process in which each work term is performed separately (through a separate change in state of the system), while all other variables are held constant. Then the overall work will be the sum of the separate work terms for the partial processes.

For a change in state of the system which involves exchange of heat, pressure-volume work, mechanical work, and chemical work, we may write an equation for the change in free energy

dG = -SdT + (-VdP) + fdl + µ_idn_i

Each of the work terms here is the product of an intensity factor (the driving force) and a capacity factor (dependent on the size of the system). The intensity factor for chemical work is the chemical potential (see below, and the other pages).

CHEMICAL THERMODYNAMICS

1) Chemical potential

The chemical potential of a system is defined by the change in free-energy of the system as a function of the change in composition. For a chemical species, i,

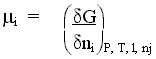
the chemical potential of i is the rate of change of free energy with change in the number of moles of i , when all other components, and work terms, are held constant.

2) Reference states

In considering chemical thermodynamics, we must talk about changes in state of the system. The system is defined by a chemical mixture of reactants and products, the change in state is a change in concentrations of the reactants as the process proceeds, and the process is defined by a chemical equation. By convention, a chemical equation is written with reactants on the left, and products on the right, and the process is assumed to occur from left to right.

It is convenient to agree on states of chemical systems to which we an refer changes. These states are:

Zero chemical potential. Complex chemicals are synthesized from the elements. The elements are assumed to have a chemical potential of zero in their natural states.

Standard states. For chemical or biochemical species in solution, the standard state is a solution of activity 1 molar (1 M). For gases, the standard state is the gas at 1 atmosphere pressure. For solvents, the activity in the pure state is arbitrarily taken as 1. This is particularly important in biochemical systems, where the aqueous solvent is assumed to have an activity of 1, which does not change significantly during a reaction. The temperature of reference states is assumed to be 25^o C unless otherwise indicated.

Standard chemical potential and chemical potential. The standard chemical potential of a species i (µ^o_i ) is its chemical potential in the standard state (an activity of 1 molar). The chemical potential at any other activity is given by:

µ_i = µ^o_i + RT ln (a_i / a_i^o)

where a_i^o is the activity in the standard state. Since the value for a_i^o is by definition 1 M, its inclusion in the above equation makes no difference to the numerical value of the logarithmic term. Inclusion does make the term in parentheses a dimensionless ratio, and this is important in understanding what's going on algebraically. However, the convention is to ignore this term, and to write the above equation as:

µ_i = µ^o_i + RT ln (a_i)