1. 
2. Help
3. Log In

Redox potentiometry

The special interest of redox reactions in thermodynamics lies in the fact that the free energy change can be measured directly using an electrochemical cell (see diagram below).

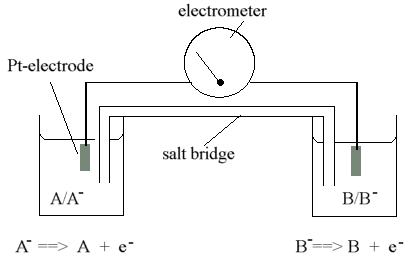
Overall reaction:

The components of the reaction are separated so that the donor couple and acceptor couple are in separate reaction vessels. The vessels are connected by a salt bridge, so that the electrostatic potential difference between them is zero. In each vessel, an electrode (usually made of Pt) connects the reaction mixture in one cell to that in the other, through the measuring device (a potentiometer or electrometer). The electrochemical cell therefore consists of two half-cells, each of which contains the reactants (oxidized and reduced components) of one redox couple, making up the half-cell reactants. In principle, the circuit allows the electrons to flow from one half-cell to the other, down the gradient provided by the difference in free energy of the electrons (measured in volts) in equilibrium with the two half-cell reactions. By channeling this flow through the measuring device, the system is constrained so that the flux is zero (or very nearly so). If a potentiometer is used, the condition of zero flux is obtained by balancing the voltage difference between the two half-cell reactions through an applied potential of opposite sign, adjusting the current, measured with a sensitive galvanometer (or other suitable device), to zero. If an electrometer is used, the voltage difference between the half-cell reactions is measured directly, the condition of zero flux being achieved by use of an electrometer of very high input impedance.

In either case, the voltage measured shows the driving force represented by the difference in free energy of the electrons in equilibrium with the two half-cell reactions. This difference is called the electrode potential difference, the oxidation reduction potential difference, or the redox potential difference.

Because the redox potential difference is measured under conditions of zero flux (the "reversible" conditions of classical thermodynamics), it is a direct measure of the free energy change of the reaction of the electrochemical cell. The electrode potential measures the intensity factor (DE, measured in volts) of the work term (DG' = -zFDE) for the process represented by the oxidation reduction reaction of the electrochemical cell (see below). In the case of the potentiometric measurement, this can be seen clearly because the voltage supplied by the potentiometer balances the electrode potential difference by application of external work from a change in state in the surroundings (the potentiometer).

The reference electrode

in which all components are in their standard states (1 atm pressure for the gas, 1M activity for the proton, or pH 0). This is not a convenient device to handle, so more often a secondary standard reference electrode is used, a calomel electrode or a Ag/AgCl electrode. These latter have redox potentials with respect to the standard H₂-electrode which are well characterized. The redox potential for a couple is usually assumed to be the value with respect to the standard reference electrode (the hydrogen elecrode). If a value is measured with respect to a secondary standard, the secondary reference electrode potential with respect to the standard is added to this value.

Free energy change for an oxidation reduction reaction

where the two half-cell reactions are those of the diagram above. We will assume that the B/B^- couple is a standard reference electrode.

 Let us consider the half-cell reaction for the couple A/A^-,

and suppose that at activities [A] and [A^-], an electrode potential of E volts is measured with respect to the reference half-cell. If the concentrations are changed to [A'] and [A' ^-], a different electrode potential of E' volts is measured. Under the conditions of measurement (zero flux), the reaction of eq. 1 is at equilibrium, in each case, with the free energy of the electron measured by the electrode potential.

or more concisely (and rearranging terms)

 Substituting from the relationship between chemical potential and concentration, we get

Let us suppose that activities A' and A' ^- were 1M, that is the standard states. Then

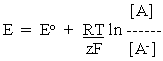-------- (2)

where E^o is the potential measured in the standard state, or the standard redox potential.

 Equation 2 is the simplest form of the equation defining the relation between redox potential (electrode potential) and the concentrations of the redox couple (or the half-cell reactants).

Practical application of redox potentiometry

Here we have the transfer of electrons and H, with the two half-cell reactions given by:

Let us consider the simple general case, represented by the half-cell reaction:

In applying eq. 2 above, we recognize that the half-cell reaction has an additional component, the H⁺. Following the same logic, we note that in the logarithmic term, we have to include all components on the oxidized side of the half-cell reaction equation in the top term of the ratio, and all components on the reduced side on the bottom.

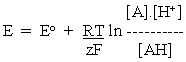--------(3)

Applying this to the half-cell reactions above, we get:

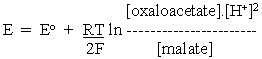
Since we want to compare electrode (redox) potentials to get an idea of the relative reducing power of redox systems, the H⁺ which crops up in these equations is an inconvenient term (see above), since most systems of biochemical interest operate near neutral pH, rather than at 1M as implied in the use of E^o. Following the same logic that we applied in making the DG equations more "useful", we transfer the energy contribution from the H⁺ concentration to the standard potential term. For the general case, eq. 3 becomes:

Applying this to the half-cell reactions of the malate oxidation above, and taking account of the stoichiometries of the protons, we get:

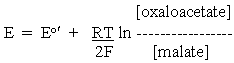
for the OAA/malate half-cell reaction, and

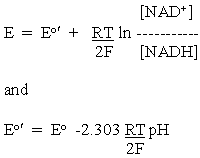
for the NAD⁺/NADH half-cell reaction.

Measuring the number of electrons and protons (H⁺) involved in a redox half-cell reaction

The equations developed above can be used to find the number of electrons and protons involved in a redox half-cell reaction, as follows:

• To find the number of electrons involved:
• Measure the electrode potential of the half-cell as the concentration ratio of oxidized and reduced components is varied. Plot ln([oxidized components]/[reduced components]) against E; the intercept (when [oxidized components] = [reduced components], or when all components are in their standard states, so that the ratio = 1) gives E^o', and the slope gives

    RT
    zF
from which z can be obtained since R and F are constants, and T can be measured.
• To find the number of protons involved:
• Measure E^o' as a function of pH, and plot results. The intercept at pH 0 gives E^o, and the slope gives

 
from which the number of protons, n, can be calculated if z is known. Since 2.303 RT/F has a value of 59.19 at 25^o C, for the couple A.H⁺/AH, n=1 and z=1, and the slope will be approx. -60 mV/pH unit; for the couple oxaloacetate.2H⁺/malate, n=2 and z=2, and the slope will also be approx. -60 mV/pH unit; for the couple NAD⁺.H⁺/NADH, n=1 and z=2, and the slope will be approx. -30 mV/pH unit.

Notes on conventions

 The standard electrode potential, E^o, is often called E_m, where the m means midpoint. This term is used because the electrode potential has the standard value when the reactants of the half-cell are poised at the midpoint between oxidized and reduced (when [oxidized components] = [reduced components]), or at the midpoint of a redox titration. For a half-cell reaction involving protons, the midpoint potential, E_m,pH=nn.nn, is often used instead of E^o', with the pH value for which the E_m value is appropriate indicated at nn.nn. The terms E_h, E_{m, pH=nn.nn} and E_m are generally used as one convention, and the terms E', E^o', and E^o, as another convention.

Relation between electrode potential and free energy of reaction