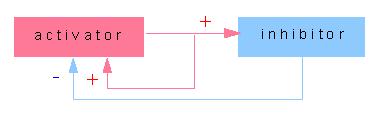
Since the mid-sixties have A. GIERER and H. MEINHARDT (Max-Planck-Institut für Virusforschung, Tübingen) dealt with the biochemical and mathematical causes of pattern formation. They were originally interested in the causes of the head induction of the freshwater polyp Hydra. It had long since been known that a new head develops only in a minimum distance to an already existing head. This is no single observation. It is a rule that no further structure (like an organ, for example) of the same kind can develop in the vicinity of an already existing one. A kind of lateral inhibition seems to be the cause. Mathematically is it explained simplest by the interaction of an activator and an inhibitor.
The activator has to fulfil two functions: it has to increase
its own activity (or synthesis), an effect called positive feedback,
and it has to force the activity (or the synthesis) of an inhibitor.
The single function of the inhibitor is to suppress the activity
of the activator. It should therefore spread through the tissue
faster than the activator, i.e. it needs a larger diffusion constant.
The diffusion constant correlates to the size of the molecule.
The smaller a molecule the more mobile is it, but a small molecule
is generally less specific than a large one. The described model
represents a control circuit. Compared to the simple regulatory
process of gradient formation is it characterized by a higher
stability (a self-amplifying effect).
Another example for control is the phototropic bending of an Avena-
coleoptile. The coleoptile bends towards a light source when just
one of its sides is exposed to light. The bending is caused by
an auxine gradient within the tissue. After the signal is removed
does the auxine gradient break down and the growth of the coleoptile
proceeds into another direction.
Lateral inhibition is very common both in the animal and the plant kingdom. The model discussed above explains the intensification - also called amplification- of the signal. Zones of high activity alternate regularly with indifferent zones. The behaviour of the postulated quantities (activator and inhibitor) can be described by two non-linear, partial differential equations:
1. delta a / delta t = rho0 rho + c rho [a2 / h] -my a + Da [delta2 h] / delta x 2
2. delta h / delta t = c rho a2 - Dh + Dh [delta2] h / delta x2
where a is the concentration of the activator and h that of the inhibitor. Both are functions of the spatial (or temporal) position. a2 is the term for the double function of the activator, 1/h that for the indicator, and my are the degradation factors (the rate of the enzymatic degradation of activator and inhibitor, respectively).
Da is the diffusion constant of the activator, Dh that of the inhibitor and rho is the frequency of the cells that produce the activator or the inhibitor. rho0 is the background production of the activator.
These differential equations are solvable. 'Suitable' values render different solutions depending on the choice of the parameters and the starting situation. Changes as a function of time can be simulated by iteration (repetitions) with the help of computers. The solutions of the equations represent structural models that mirror the spatial and temporal pattern development.
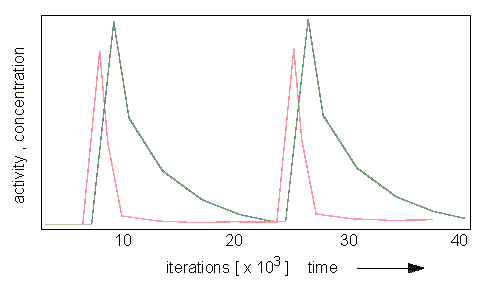
Oscillating Distribution of Activator (red) and Inhibitor (grey). (H. MEINHARDT and A. GIERER, 1974).
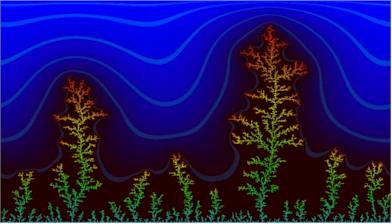
It is possible to calculate parameters that are necessary for the development of stable patterns and such that cause unstable (statistical) distributions (H. MEINHARDT and A. GIERER, 1974). In that way can, for example, the development of a leaf primordium during the shoot growth of a plant be simulated. The distribution of cells or organs within a two-dimensional field as in the case of guard cell distribution or hairs at the surfaces of leaves is a possible application, too. The statistical (stochastic) distribution is always caused by a local instability of the activator / inhibitor equilibrium.
The growth is simulated by the doubling of the cells at the upper end of the cylinder. The position of the first maximum is accidental. It determines the position of all following maxima. The maxima can be arranged at certain angles (60°, 90°, 120°, etc.) and distances (alternate leaves) or in angles of 180° (opposite; H. MEINHARDT, 1974).Regular periodic structures emerge if the pattern formation works during growth. (a) an alternating (distichous) pattern of leaf initiation is simulated by assuming a cylinder that grows at its upper end. New maxima appear in 180° arrangement. (b) If the diameter of the cylinder is somewhat larger and/or the range of the inhibitor is shorter, pairs of maxima at opposite sides. Each subsequent pair is displaced by 90° (decussate pattern). The existence of helical arrangements of leaves suggest a more complex mechanism (see Fig. 19-23 ).
What's the point of all that? Models like these give valuable information when looking for morphogenic inductors. Computer models can be calculated until the wanted aim is achieved and the model mirrors the natural structure as good as possible. The calculations have to start with the size of the organ primordia, the number and size of the cells, etc. If these values are available, the diffusion constants of activator and inhibitor can be calculated. The diffusion constant gives a clue about the molecular weights of activator and inhibitor that can be taken into consideration when trying to find the substance with biochemical methods.
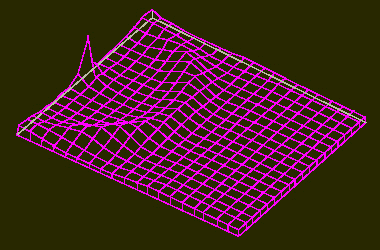
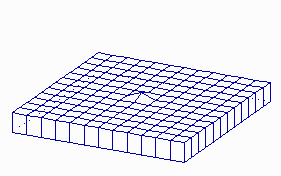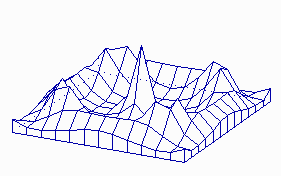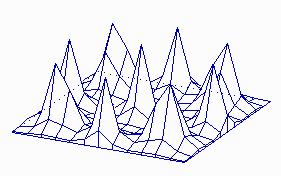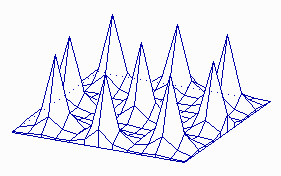
Maxima of an Activator within a Two-Dimensional Field (like a leaf surface).
a. The system is started by a surplus of activator at one position within the field. A ring-shaped zone with a high activator concentration results. Its distance from the starting position is larger than the range of the inhibitor.
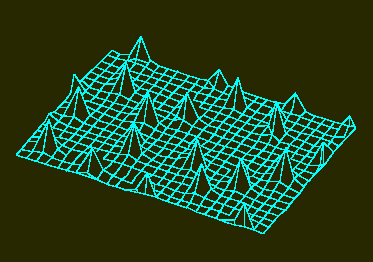
b. The structure is unstable however and disintegrates into a number of single activator maxima. These maxima develop at certain distances from each other. Their distribution is accidental. Such a pattern develops in a non-growing field with small statistical fluctuations of the activator concentration in the starting phase (H. MEINHARDT, 1974)
Leaf venation is another example for pattern formation. The picture at the right shows
the leaf veins of a tropical fern (cf. Asplenium). The
preparation macerated under natural conditions. Its parenchyma
and epidermal cells have decomposed and only the xylem-containing
elements remained.
Usually branch side veins off at certain angles from a main vein. The side veins are again starting points of further ramifications. How does such a network develop and why are certain basic patterns kept despite species-specific differences?
H. MEINHARDT (1976) proposed as an explanation a rather simple mechanism that is based on a development of the theory of pattern formation just discussed. It requires a high local concentration of an activator (A) and an additional inhibitor (I). It is assumed that the inhibitor concentrates at the margins of growing leaves . The activator is a signal for cell differentiation. The differentiated cell adopts new properties that differ from those of an undifferentiated cell. It is assumed to suppress the production of the activator and to repel the signal that led to its production. As a consequence is the activator produced in the neighbouring cell and causes this cell to differentiate, too. The two cells do subsequently contact each other and initiate thus the development of a cell row. The suppression of the activator production causes the activator's production centre to be pushed forward by the steadily elongating cell row
In order to produce a network are not only ramifications but backward connections essential, too. For this is a third component - S - with a control function required. It is assumed that S is ubiquitous in tissues and that it is destroyed by a growing cell row so that its concentration decreases around every differentiated cell. Under the further assumption that S stimulates the activator production drifts the activity maximum to zones with high concentrations of S. As a consequence develop cell rows mainly in the middle of a tissue and avoid peripheral areas. After a row has elongated by a certain amount is the inhibiting effect (the destroying of S) of the tip not sufficient any more to suppress weak maxima of activity that develop along the finished row. Such weak maxima occur therefore mainly in areas with a high local concentration of S where they induce ramifications. A second ramification can evolve only at a sufficiently large distance from the previous one. The development of rows is characterized by the attempts to maintain a distance from the margin. Such a developing network is marked by a high stability. If, for example, all differentiated cells of one of its halves are removed, then the network will regenerate. The new part would resemble the pattern of the original one but not be alike. Most ramifications end blind and backward connections are rare - both in the model and in nature. They occur occasionally and are best explained by an interaction of the two inhibiting factors (decrease of S, increase of I). A decrease of S along an existing row has the weaker effect so that a growing and an existing row repel each other far less than two rows that grow towards each other.
What would happen if the model omitted S? It would then produce dichotomous ramifications and backward connections would be impossible. As a consequence are the developing structures mechanically unstable. Dichotomous ramifications of leaf veins developed earlier during evolution than complex networks. They occur, for example, among pteridophytes and gymnosperms while complex networks are typical for angiosperms.
The theory of pattern formation according to GIERER and MEINHARDT that we have just discussed demonstrates how zones of different activities develop in originally uniform fields by the formation of stabilized gradients. It does not explain the further development of areas into activity maxima or minima. The distribution of activity maxima shown in the picture above could be a description of the development of a hair pattern at the surface of an insect. It could, too, describe the distribution pattern of stigmata at a leaf's undersurface. It applies equally to leaf venation and to the ramification type of blood vessels within a vertebrate.
It can be assumed that the regular patterns underlying a plant's development and its phenotype are the result of the smallest possible number of decisions. This assumption led to the development of a number of model concepts (LINDENMEYER, 1971, 1975, 1984 or FRITJERS, 1978 among others). All models work with a small number of modules (repeating units), combinations of modules, iterations of modules and iterations of sets of modules.
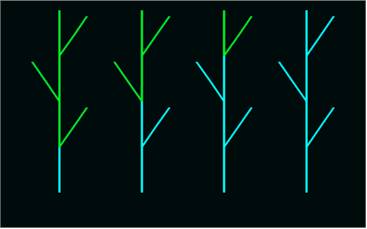
Plant Shoot Organized from Modules. A signal (blue) spreads from basis to tip, i.e. in an acripetal direction.
LINDENMEYER (1971, 1975) defined morphogenesis at the molecular level as the aggregation of macromolecules to larger structures like membranes, microtubuli or flagella. The aggregates themselves determine the shape of cells and organelles. Moreover do they have an influence on the direction of cell division, on cell enlargement and on cellular transport processes. Groups of cells act like units, they form tissues and organs. In this way is finally a description of the growth processes of the complete organism at a morphological level possible. A complete description of a developing organism would have to take into account the essential processes and structures in the right succession at all organisational levels. Since cells are the only autonomous units with genetic continuity in all higher organisms, are they, too, the elementary units of all developmental processes. The main problem about it is to find out what kind of instruction is required in order to change their time- and space-dependent constellation, i.e. to stimulate them to divide, to enlarge, to wither away, or to differentiate. There exists no definite answer to that since the required algorithms can manifest themselves in different ways. Besides can other algorithms lead to the same result. The unravelling of a set of instructions is therefore no exclusive explanation of a developmental process.
LINDENMEYER's model of a multicellular organism consists of a succession of symbols, in which each symbol represents a cell whose development follows algorithmic rules that allow a substitution of symbols. Each symbol follows its own rules. Substitutions express processes like cell divisions, death or changes in the state of differentiation. They can be influenced by neighbouring cells (dependence on cell-cell-interactions) or by the state of the cell itself (development without cell-cell-interactions). The repeated use of a set of algorithms can thus simulate a sequence of developments. Additional control mechanisms modulate the described processes so that a hierarchy of sequences is the result. The model serves mainly to interpret the behaviour of cell lineages and to describe their status within the whole system. In principle can each cell change its status repeatedly. It can, for example, divide equally or unequally. This allows to design models resembling a leave with an entire or a subdivided margin. Hereby the concept is founded on the assumption that all cells of a leaf develop from one row of cells within the leaf primordium. Only the cells of the developing leaf margin have to be regarded in order to generate a leaf shape. The model requires just five cellular states (repeated units) to explain the shape of a compound leaf.
A model that takes additionally cell-cell interactions (transport of molecules, diffusion of activating substances) into account is far more flexible and explains thus more complex shapes, too. It evens out fluctuations better (see also network generation according to GIERER and MEINHARDT). The shapes generated by such an algorithm resemble each other but they are not alike. It is possible to integrate extern factors like light, humidity, temperature, periodic quantities (day length), and others into the model.
In 1978 presented FRITJERS a concept for the interpretation of the ramification pattern of inflorescences. Each inflorescence consists of a set of modules: internodes, nodes, bracts, vegetative apical meristems and flowers. In addition are switches like the transition from an apical meristem to an internode, a node, leaves or a flower necessary to explain the development of an inflorescence. An elementary example will serve as an illustration. The apical meristem is symbolized by A. The development of an internode plus one flower shall be defined as follows:
A > I (F) A
i.e. an internode, a flower (that ceases to develop further), and a new apical meristem develop. The following model shows that this instruction leads to a simple inflorescence with seven ramifications after seven successive steps.
0. A
1. I (F) A
2. I (F) I (F) A
3. I (F) I (F) I (F) A
4. I (F) I (F) I (F) I (F) A
5. I (F) I (F) I (F) I (F) I (F) A
6. I (F) I (F) I (F) I (F) I (F) I (F) A
7. I (F) I (F) I (F) I (F) I (F) I (F) I (F) A
An inflorescence with a terminal flower develops, if a further stimulus (an additional switch) that induces the transformation of A > F is introduced:
0. A
1. I (F) A
2. I (F) I (F) A
3. I (F) I (F) I (F) A
4. I (F) I (F) I (F) I (F) A
- switch -
5. I (F) I (F) I (F) I (F) I (F) (F)
One or several further factors modulate the flower size or the length of the internodes, for example. As a result could the units decrease with each step, or a shape with small bottom and top and larger middle units could develop.
Such modulating factors may be hormones whose spreading through tissues can be explained by gradients. Switches represent active or inactive genes whose activities are required for the development of the structure in question. One group of genes with a regulating function is known: the so-called homeotic genes, whose activity influences that of other genes. In this way can they activate numerous further genes at a lower hierarchical level, like a set of genes required for the development of a flower or a leaf. Homeotic genes are thus on a high hierarchical level. Their switch function determines the generation of specific morphological structures.
Acripetal and Basipetal Spreading of a Signal. In the first case develop flowers and fruits from the bottom of the inflorescence first, in the second case occurs differentiation from the tip to the basis.